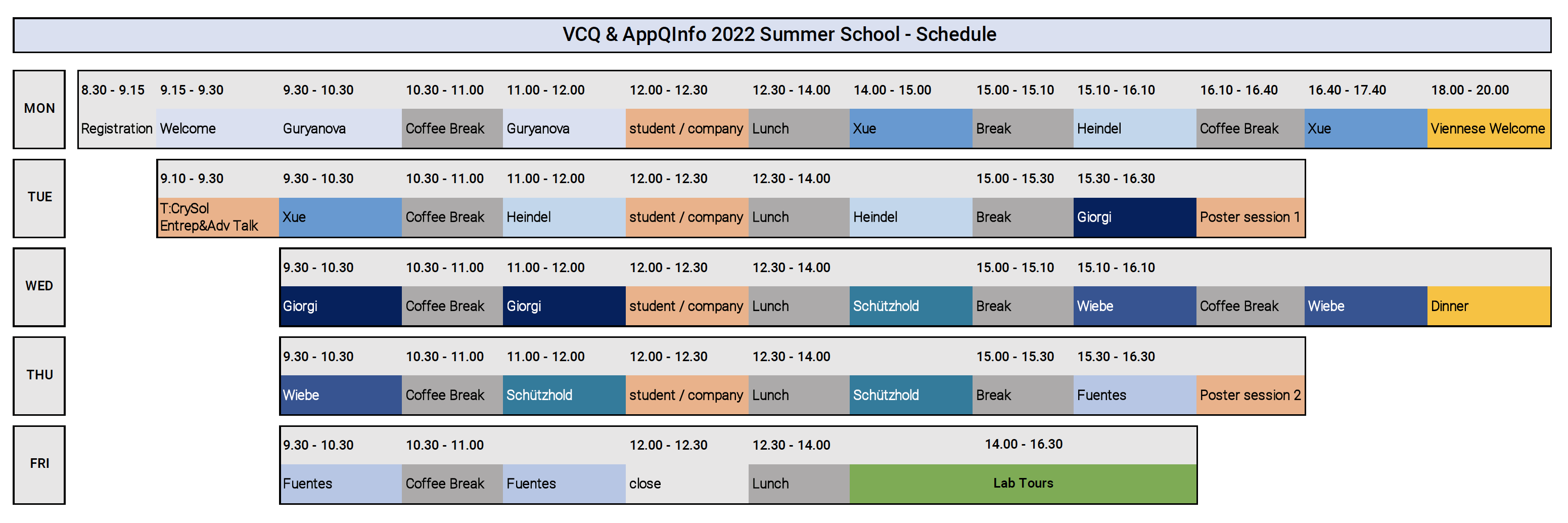
VCQ Summer School 2022 Program

VCQ Summer School 2022: Program

HOME
REGISTRATION
VENUE

Relativistic Quantum Information and Metrology

The insight that the world is fundamentally quantum mechanical inspired 
the development of quantum technologies including quantum computation, 
communications and metrology. However, the world is not only quantum but 
also relativistic, and indeed many implementations of quantum 
information tasks involve truly relativistic systems.
In this lecture series I consider relativistic effects on entanglement 
in flat and curved spacetimes. I will emphasize the qualitative 
differences to a non-relativistic treatment, and demonstrate that a 
thorough understanding of quantum information theory requires taking 
relativity into account. The exploitation of such relativistic effects 
will likely play an increasing role in the future development of quantum 
technologies. The relevance of these results extends beyond pure quantum 
information theory, and applications to quantum metrology and to 
foundational questions in cosmology and black hole physics will be 
presented.
Reservoir Computing (RC) and extreme learning machines are machine-learning paradigms that make use of the information processing capability of complex dynamical systems [1]. Recently, quantum systems have been suggested as promising candidates for reservoir computing due to the significant growth in phase space degrees of freedom [2].

In this lecture, I will first introduce the general concepts and working principles of reservoir computing in the context of artificial neural networks, and then I will define the properties and minimal ingredients that physical systems need to satisfy to be employed as reservoir computers. Then, I will motivate the use of quantum systems for reservoir computing and describe the existing theoretical and experimental approaches [3].

Special attention will be devoted to the physics behind quantum reservoir computing, in particular regarding the phenomenon of dynamical phase transitions [4]. Another fundamental issue tackled in this lecture will be represented by the role of quantum measurements in RC, the limitations they introduce, and the possible way to overcome these limitations.
 
[1] H. Jaeger, The echo state approach to analysing and training recurrent neural networks-with an erratum note, German Natl. Res. Center Inf. Technol. GMD Tech. Rep. 148, 13 (2001); W. Maass, T. Natschläger, and H. Markram, Real-time computing without stable states: A new framework for neural computation based on perturbations, Neural Comput. 14, 2531 (2002).
[2] K Fujii and K Nakajima, Harnessing disordered-ensemble quantum dynamics for machine learning. Physical Review Applied, 8, 024030 (2017).
[3] P Mujal, R Martínez-Peña; J Nokkala; J García-Beni; GL Giorgi; MC Soriano, R Zambrini. Opportunities in Quantum Reservoir Computing and Extreme Learning Machines. Advanced Quantum Technologies. 4, 2100027 (2021).
[4] R Martínez-Peña, GL Giorgi, J Nokkala, MC Soriano, R Zambrini, Dynamical phase transitions in quantum reservoir computing, Physical Review Letters 127, 100502 (2021).

In recent years, tremendous progress has been achieved in the engineering of solid-state-based quantum light sources. In this context, semiconductor quantum dots (QDs) are among the most promising candidates for implementations of quantum information [1]. In my talk, I will review our progress in this field, ultimately striving towards quantum networks at global scales. I will discuss the development of novel building blocks, including fiber-pigtailed quantum devices [2,3] and plug&play benchtop single-photon quantum key distribution (QKD) systems [4], as well as spin-photon interfaces [5]. I show how to optimize and certify the performance of QKD systems [6] and report on our recent efforts in transferring our knowledge to advanced protocols and different technology platforms, including quantum emitters in 2D materials [7]. Assembling these building blocks to functional multi-partite quantum networks is a grand challenge in quantum technologies which will be tackled in my group.

[1] D. A. Vajner et al., Advanced Quantum Technologies 2100116, Invited Review Article (2022)

[2] L. Rickert et al., Optics Express 27, 36824 (2019)

[3] L. Rickert et al., Applied Physics Letters 119, 131104 (2021)

[4] T. Gao et al., Applied Physics Reviews 9, 011412 (2022)

[5] T. Heindel et al., APL Photonics 2, 121343 (2017)

[6] T. Kupko et al., npj Quantum Information 6, 29 (2020)

[7] T. Gao, M. von Helversen, C. Anton-Solanas, C. Schneider, and T. Heindel, Atomically-thin Single-photon Sources for Quantum Communication, arXiv:2204.06427 (2022)

After a general introduction into quantum simulators, this lecture will be devoted to simulations of fundamental phenomena. To this end, the underlying basic principles of various fundamental effects will be introduced briefly. Afterwards, several laboratory systems will be discussed which display analogous effects — in the spirit of the famous quote from Richard Feynman:

“The same equations have the same solutions.”

In this talk I will review the topic of quantum simulation and show how these algorithms can be used in order to solve important problems in chemistry and quantum field theory.  In particular, I will review simulation of chemistry using first and second quantization and show how algorithms such as Trotter-Suzuki algorithms and qubitization can be used to solve important simulation problems.  Finally I will discuss how such ideas can be hybridized and algorithms that divide up the quantum simulation problem over different algorithms based on the features of the part of the molecule that we wish to simulate.

In this lecture, I will first describe the basics of quantum dots and spin qubits in a semiconductor. Then I will discuss the recent progress in experimental quantum information processing with spin qubits and the application of quantum dots in quantum simulations.